Navigating the world of decimals and fractions can often seem daunting, especially for our budding 6th, 7th, and 8th mathematicians. How do you convert decimal to fraction without a calculator? What’s the trick to turning repeating decimals into readable fractions? If these questions resonate with you, you’re in for a treat. In our worksheets, we’ll explore how to seamlessly convert decimals into mixed and improper fractions, delve into the specifics of tenths, hundredths, and thousandths, and master the art of converting those tricky repeating decimals.
And the best part? We’ll provide 20 free printable worksheets aligned with Common Core standards for hands-on practice. Each sheet is tailored for optimal understanding, complete with an answer key. So, whether you’re an educator searching for quality resources or a student eager to practice, these worksheets are your ticket to mastering the conversion process. Dive in, and let’s demystify the magic of converting decimals to fractions together!
How to Convert Decimals to Fractions: A Comprehensive Guide
Starting your journey into math, especially when delving into fractions and decimals in the 6th, 7th, and 8th grades, can seem a tad overwhelming. However, fret not. Things flow more smoothly once you get the hang of the basics.

The foundation of this guide lies in understanding the core elements of decimals. So, let’s kick off with the fundamental divisions: tenths, hundredths, and thousandths. Imagine you’re slicing a pizza. When you divide it into ten equal slices, each piece represents a tenth. Similarly, split a single slice into ten tiny slivers, each representing a hundredth of the whole pizza. And going even further, if you were to divide one of those slivers into ten minute portions, each would be a thousandth. These divisions manifest as places after the decimal point in decimals, making conversion a systematic process.
Converting Without a Calculator: Hands-On Practice for Middle Schoolers
Now, armed with the basics, let’s delve deeper into a more hands-on approach, especially for our eager 6th to 8th graders. One might wonder, “Why learn conversions without a calculator?” Well, the beauty of math lies not just in finding the answer but in understanding the process. Let’s admit it: there’s a particular pride in solving problems manually!

With the Common Core Standards, there’s an emphasis on conceptual understanding. So, while free printable worksheets that align with these standards can be a fantastic resource, first, grasp the steps:
- Identify the place value of the last digit of the decimal.
- Convert the decimal into a fraction using the place value as the denominator.
- Simplify the fraction if necessary.
Mastering Mixed and Improper Fractions: Conversion Techniques
As we journey further, we encounter mixed and improper fractions. Both sound complicated. Yet, with a few strategies up your sleeve, these become another exciting challenge to conquer.

As the name suggests, a mixed fraction is a mix – a whole number and a fraction combined. On the other hand, an improper fraction has a numerator that’s larger than its denominator. Converting decimals to either of these fractions follows a straightforward approach.
For instance, for a decimal like 1.75, one can observe that there’s a whole number, 1, and a decimal, .75. To convert the decimal part, we follow the earlier steps, resulting in 75/100, which simplifies to 3/4. So, 1.75 becomes the mixed fraction 1 3/4.
Tackling Repeating Decimals: Converting to Fractions Simplified
Now, here’s a curveball: repeating decimals. You’ve seen them – numbers like 0.666… or 0.121212… that seems to go on forever. But even these tricky numbers have a systematic way of conversion.
For repeating decimals, a neat trick is to use algebra. First, let’s label our decimal as x. Using the example of 0.666…, we can represent it as x = 0.666…
Multiplying both sides by 10 (or 100 or 1000, depending on the repeating pattern’s length) will shift the decimal point. In our example: 10x = 6.666…
Subtracting the original equation from this gives 9x = 6, simplifying to x = 6/9 or 2/3 when reduced.
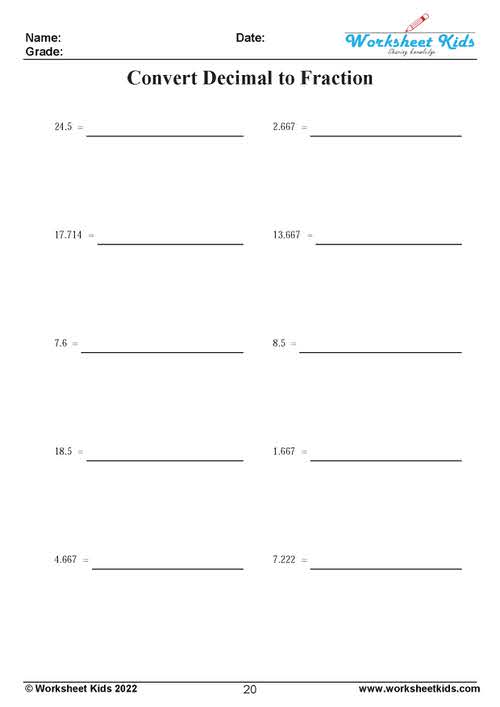
It’s magic. Not entirely, but it’s the beauty of understanding the intricacies of numbers.
Get Ahead with Practice: Free Worksheets for Decimal to Fraction Conversion
As the adage goes, “Practice makes perfect.” And it’s especially true in math. Now that you know the various techniques of converting decimals to fractions, it’s time to put that knowledge into practice.

Many free printable exercises are tailored for 6th to 8th-grade levels. Intricately designed and aligned with the Common Core Standards, these worksheets can be your trusty companions on this math adventure. Moreover, these sheets come complete with an answer key, making self-assessment a breeze.
In conclusion, the journey of converting decimals to fractions, from the basic tenths to the complex repeating decimals, is filled with discoveries and aha moments. As you transition from one grade to another, you’ll find that with every worksheet, every challenge, and every fraction you simplify, you’re not just learning numbers – you’re mastering a language, the universal language of math.